Pattern of Studies

● Interactive Classes
● Home Assignments
● Class Notes
● Periodic Class Tests
● Student's Monthly Report
● One to One Attention
● Doubt Classes
● Syllabus Revision

Report Analysis Parameters

● Tests Marks
● Attendance
● Class Discipline
● Class Response
● Home Assignments
● Student's Comparison with Class
● Last 3 Months Performance
● Special Remarks

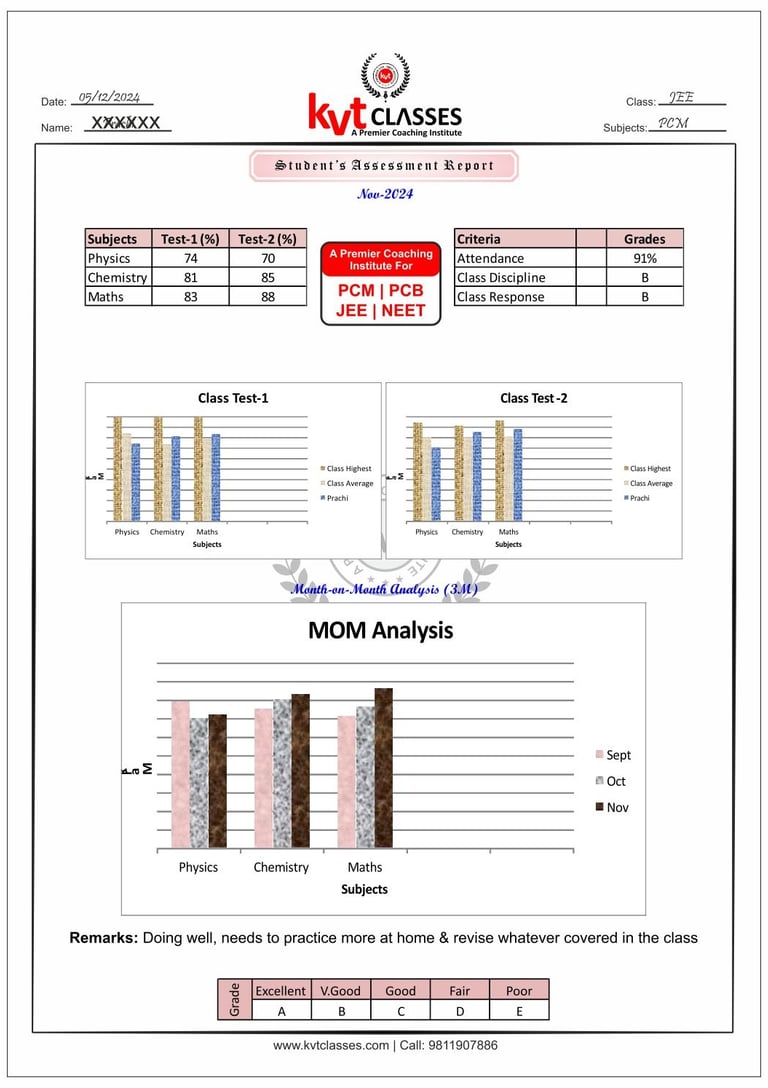
Student's Sample Report

Class Tests

● Two tests per subject in a month
● Test schedule on Saturday in extra hours
● Result within 1 week of test conduct
● Test discussion on result day
● Mistakes rectification briefing

Conduct of Classes

● On board explanation with notes
● Make Concept clear with practical examples
● Interactive class sessions
● Home work / Assignment at the end of the class
● Home work / Assignment check in the next class
● Quick recap of previous class in the next class
● Two tests per month/subject on Saturdays
● Class duration 1.5 Hr/sub alternate days (4.5Hrs./wk)
● Extra classes provided when needed

Classes Schedule 2024-25

JEE for Class XI / XII / Dropper Batch

Note: Class Tests will be conducted on Saturday in extra hours

Preparation Tips for JEE

Preparing for the Joint Entrance Examination (JEE) requires a well-structured plan, dedication, and consistent effort. Here are some preparation tips to help you excel in JEE:

1. Understand the Exam Pattern and Syllabus

Understand the exam pattern (number of questions, marking scheme, etc) for JEE Main and Advanced.
JEE Main: Focus on Physics, Chemistry, and Mathematics (equal weightage).
JEE Advanced: More conceptual and analytical questions.
Syllabus: Thoroughly go through the NCERT syllabus for Class 11 and 12, as it forms the foundation.

2. Create a Study Plan

Daily Schedule: Allocate time for each subject daily.
Weekly Goals: Set achievable targets for each week.
Revision Time: Dedicate time for regular revision.
Mock Tests: Include regular practice tests to assess your progress.

3. Focus on Conceptual Clarity

Physics: Understand concepts and practice numerical problems.
Chemistry: Focus on both theory (Inorganic) and problem-solving (Physical and Organic).
Mathematics: Practice regularly to improve speed and accuracy.

4. Use the Right Study Material

Books:
Physics: HC Verma, DC Pandey
Chemistry: NCERT, OP Tandon, MS Chauhan
Mathematics: RD Sharma, Arihant, ML Khanna

5. Practice Previous Years’ Papers

Solve at least 10 years of JEE Main and Advanced papers.
Analyze your mistakes and work on weak areas.

6. Take Mock Tests

Simulate exam conditions to improve time management.
Analyze your performance and identify areas for improvement.

7. Strengthen Your Basics

Focus on NCERT books for Chemistry and Mathematics.
Build a strong foundation in Physics concepts.

8. Time Management

Allocate time wisely during the exam.
Practice solving questions within a set time limit.

9. Stay Healthy and Positive

Sleep: Get 6-8 hours of sleep daily.
Diet: Eat healthy and stay hydrated.
Exercise: Take short breaks and engage in physical activities to stay fresh.

10. Stay Consistent and Motivated

Avoid procrastination.
Stay focused on your goal and maintain a positive mindset.

11. Seek Help When Needed

Clear doubts with teachers, mentors because concept clarity is the key.
KVT Classes help you clear every concept, but self-study is equally important.

12. Revision is Key

Regularly revise formulas, concepts, and important topics.
Make short notes for quick revision.

13. Avoid Common Mistakes

Don’t ignore any subject or topic.
Don’t rely solely on shortcuts; focus on understanding.
Avoid last-minute cramming.

14. Stay Updated

Keep track of exam dates, admit cards, and any changes in the exam pattern.

UNIT 1: SETS, RELATIONS AND FUNCTIONS:

Sets and their representation; Union, intersection and complement of sets and their algebraic properties; Power set; Relations, type of relations, equivalence relations, functions; one-one, into and onto functions, the composition of functions.

UNIT 2: COMPLEX NUMBERS AND QUADRATIC EQUATIONS:

Complex numbers as ordered pairs of reals, Representation of complex numbers in the form a + ib and their representation in a plane, Argand diagram, algebra of complex numbers, modulus and argument (or amplitude) of a complex number, Quadratic equations in real and complex number systems and their solutions; Relations between roots and coefficients, nature of roots, the formation of quadratic equations with given roots.

UNIT3: MATRICES AND DETERMINANTS:

Matrices, algebra of matrices, type of matrices, determinants and matrices of order two and three, evaluation of determinants, area of triangles using determinants; Adjoint and inverse of a square matrix; Test of consistency and solution of simultaneous linear equations in two or three variables using matrices.

UNIT 4: PERMUTATIONS AND COMBINATIONS:

The fundamental principle of counting, permutations and combinations; Meaning of P(n, r) and C(n, r). Simple applications.

UNIT 5: BINOMIAL THEOREM AND ITS SIMPLE APPLICATIONS:

Binomial theorem for a positive integral index, general term and middle term and simple applications.

UNIT 6: SEQUENCE AND SERIES:

Arithmetic and Geometric progressions, insertion of arithmetic, geometric means between two given numbers, Relation between A.M and G.M.

UNIT 7: LIMIT, CONTINUITY AND DIFFERENTIABILITY:

Real–valued functions, algebra of functions; polynomial, rational, trigonometric, logarithmic and exponential functions; inverse functions. Graphs of simple functions. Limits, continuity and differentiability. Differentiation of the sum, difference, product and quotient of two functions. Differentiation of trigonometric, inverse trigonometric, logarithmic, exponential, composite and implicit functions; derivatives of order upto two, Applications of derivatives: Rate of change of quantities, monotonic-Increasing and decreasing functions, Maxima and minima of functions of one variable.

UNIT 8: INTEGRAL CALCULAS:

Integral as an anti-derivative, Fundamental integrals involving algebraic, trigonometric, exponential and logarithmic functions. Integration by substitution, by parts and by partial fractions. Integration using trigonometric identities.
The fundamental theorem of calculus, properties of definite integrals. Evaluation of definite integrals, determining areas of the regions bounded by simple curves by simple curves in standard forms.

UNIT 9: DIFFRENTIAL EQUATIONS :

Ordinary differential equations, their order and degree, the solution of differential equation by the method of separation of variables, solution of a homogeneous and linear differential equation

UNIT 10: CO-ORDINATE GEOMETRY :

Cartesian system of rectangular coordinates in a plane, distance formula, sections formula, locus and its equation, the slope of a line, parallel and perpendicular lines, intercepts of a line on the co-ordinate axis.
Straight line: Various forms of equations of a line, intersection of lines, angles between two lines, conditions for concurrence of three lines, the distance of a point form a line, co-ordinate of the centroid, orthocentre and circumcentre of a triangle.
Circle, conic sections: A standard form of equations of a circle, the general form of the equation of a circle, its radius and centre, equation of a circle when the endpoints of a diameter are given, points of intersection of a line and a circle with the centre at the origin and sections of conics, equations of conic sections (parabola, ellipse and hyperbola) in standard forms.

UNIT 11: THREE DIMENSIONAL GEOMETRY :

Coordinates of a point in space, the distance between two points, section formula, direction ratios and direction cosines and the angle between two intersecting lines. Equation of a line; Skew lines, the shortest distance between them and its equation.

UNIT 12: VECTOR ALGEBRA :

Vectors and scalars, the addition of vectors, components of a vector in two dimensions and three-dimensional spaces, scalar and vector products.

UNIT 13: STATISTICS AND PROBABILITY :

Measures of dispersion; calculation of mean, median, mode of grouped and ungrouped data, calculation of standard deviation, variance and mean deviation for grouped and ungrouped data. Probability: Probability of an event, addition and multiplication theorems of probability, Baye's theorem, probability distribution of a random variable.

UNIT 14: TRIGONOMETRY :

Trigonometrical identities and trigonometrical functions, inverse trigonometrical functions their properties.

Detailed Syllabus JEE (Main) Mathematics